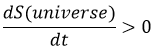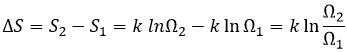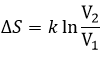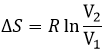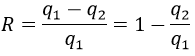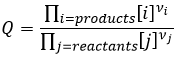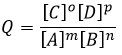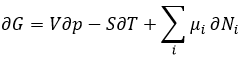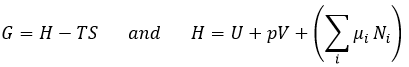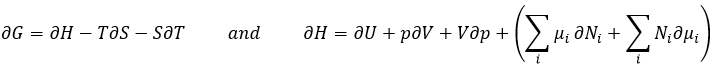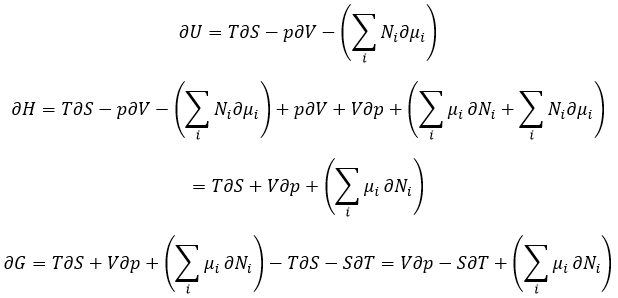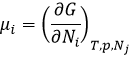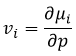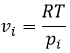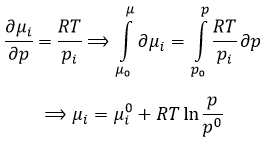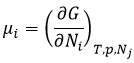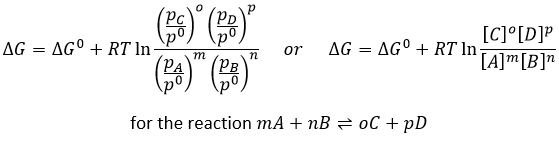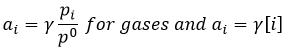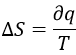Second principle
Increase of the entropy: The entropy in the universe increases over time.
The entropy is a measure of the disorder of a system. The second law of thermodynamics has several formulations but the most common is that a process is spontaneous if, without external influence (isolated system), it induces an increase of the entropy in the universe.
For instance, if two reservoirs are connected, one being empty and the other one full of gas, the gas will spontaneously occupy the whole system even if it does not involve any variation of energy.
The gas dilates because there are more positions to occupy in a larger volume. Another definition of the entropy is that a system tends to change to the most probable state.
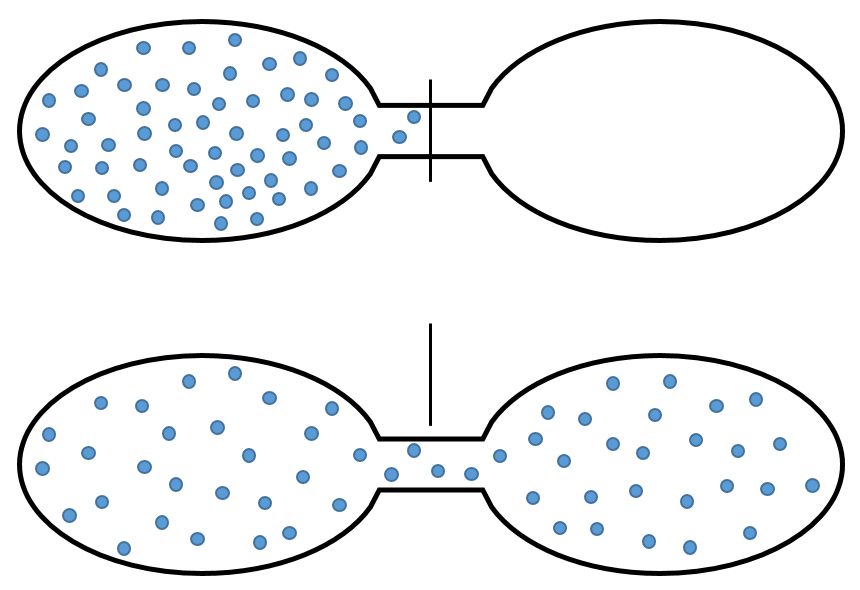
Imagine a small box with only a few available positions. Two particles are in this box and each one occupies one spot. Several combinations are possible:
If we increase the volume of the box, the amount of possibilities increases exponentially. It is an entropy of position/disposition. The formula of this kind of entropy for one particle is
Ω being the amount of possible configurations for this particle.
If the volume containing this particle changes from a volume V1 to a volume V2, the variation of entropy is
The number of positions for one particle is directly proportional to the volume and
For diluted gases, the volume of the particles is negligible with regard to the volume of the system. As a result, we can barely ignore the presence of other particles in the formula and say that for NA particles (1 mole)
We have seen earlier the dilatation of a gas that the energy was a state function but that the work and the heat were not and thus that the way the process takes place has an influence on the work and on the heat. The third path we discussed was the isothermal process for which we found an expression for the work
This process is isothermal so ΔE=q+W=0.
We can thus find the variation of entropy for this process from the heat. If the gas dilates, heat enters the system (it is an endothermic process).
The variation of entropy is thus equivalent to the quantity of heat that the gas has to absorb to keep its temperature constant.
The states of the matter don’t have the same entropy. A gas has an entropy way larger than a liquid, itself a bit larger than the one of a solid. We can easily understand that using the repartition of particles in a small box.
In a (perfect) gas, the particles don’t have interactions between them. They can thus occupy any position of the volume without restriction. In a liquid, the particles interact together and stay grouped but the shape of the cluster is not fixed. The possibilities are thus more limited than for a gas. In a solid, the particles are fixed together in a given shape. The positions that a solid can take are thus more limited than the ones that a liquid can take and way more limited than for a gas.
Tables of standard entropies exist and give the entropy of molecules in a given state at 298K.
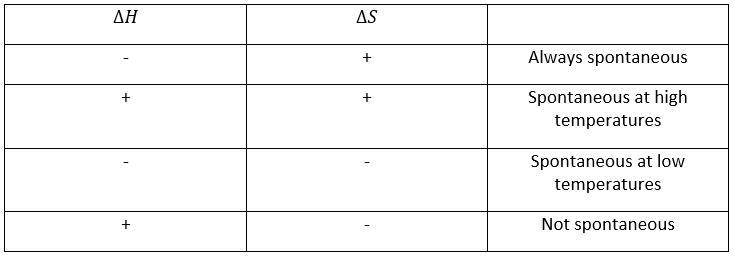
To resume, several cases can be distinguished. A process occurs if the production of entropy in the universe is positive. This production of entropy is composed of the variation of entropy in the system and in the environment. One of those components of the entropy can be negative if the other component is positive and larger in absolute value.
When a reaction produces a gas, the variation of entropy is positive. If this reaction is exothermic, heat is transmitted to the environment, meaning that its entropy increases. This reaction is thus spontaneous. If the reaction was endothermic, the variation of entropy of the environment would have been negative. The reaction could still be spontaneous if the production of entropy of the reaction is larger than the decrease of entropy in the environment.
Cycle of Carnot
Nicolas Léonard Sadi Carnot proposed a way to convert thermal energy into work. It is a thermodynamic cycle, i.e. a series of transformations of state leading back to the initial state, composed of two isothermal processes (no temperature variation) and two adiabatic processes (no heat exchange with the environment). All the processes are reversible and the cycle can thus be done in the opposite way, converting work into a difference of temperature.
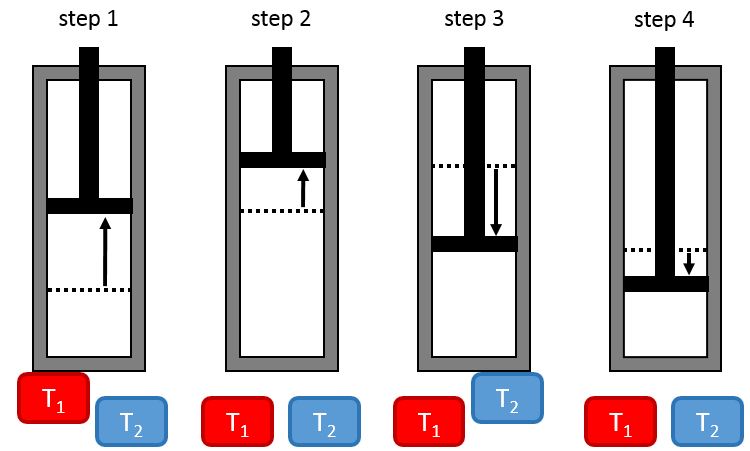
The setup is made of one reservoir the volume of which can change and that can be in contact with two bodies of temperature T1 and T2, T1 being larger than T2.
First step: isothermal expansion of the gas at T1. The reservoir is in contact with the hot body at T1. Heat q1 is exchanged between the body and the reservoir of gas that expands without variation of temperature. There is thus a variation of entropy ΔS1=q1/T1.
Second step: adiabatic expansion of the gas. During this second step there is no exchange of heat but the gas continue to expand, doing work on the piston and cooling from T1 to T2. The body at T=T1 is not in contact with the reservoir anymore. As there is no exchange of heat, there is no variation of entropy.
Third step: isothermal compression of the gas at T2. The reservoir is now in contact with the second body, at T=T2, causing a transfer of heat q2 while a compression is applied by the piston, keeping the temperature constant. There is thus a variation of entropy ΔS2=q2/T2.
Fourth step: adiabatic compression of the gas. The body at T=T2 is not in contact anymore and there is no possible heat transfer. The gas continue to decrease in volume while its temperature changes from T=T2 to T=T1. At the end of this step, the system is back in the conditions (volume, temperature and pressure) of the initial state.
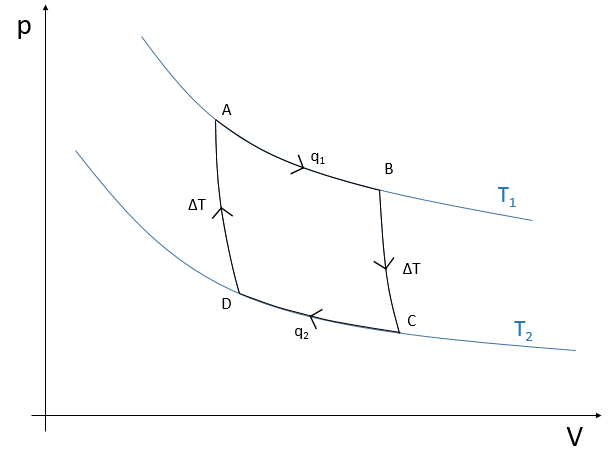
The cycle can be plotted in the pV phase diagram as shown below:
The efficiency R=W/q1 of the cycle of Carnot would be 100% if all the work W was transformed into heat q. The work here is the difference of heating q1-q2 because ΔE=0=q+W (isolated system, the bodies at T1 and T2 being in the system). As a result,
It is possible to show that the ratio q2/q1 is equal to the ratio T2/T1.
The consequence is that the efficiency is never at 100% except at T2=0K, that we can’t reach on earth. The loss of energy is caused by the production of entropy.
Free energy of Gibbs
For a reaction to occur, we just saw that two factors are important: the enthalpy of reaction (if the reaction is exo or endothermic) and the variation of entropy. The free energy of Gibbs ΔG regroups the two factors in one equation
A reaction is spontaneous if ΔG<0. The entropic term clearly shows that a reaction can be spontaneous at a given temperature but not if the temperature is modified
We can find tables for the values of the standard values of ΔH0 and ΔS0 and subsequently tables of ΔG0 for reactions of formations of molecules. At other temperatures but at 1atm, the relation is
Q is the reactive quotient that we already met previously
For instance, the reactive quotient of this reaction
Is
If ΔG is negative, the reaction goes towards the right, if it is positive, the reactions goes to the left. If ΔG=0, we are at the equilibrium. As a result, we can find a relation connecting the constant of equilibrium K with the free energy of Gibbs:
The chemical potential
The chemical potential μi is the energy of a molecule i that can be used during a reaction or a physical process.
Equation of Gibbs-Duheim:
The last term is thus a variation of energy in the system due to a variation of the quantities of particles in the system, something that we did not take into account before (it will be shown between brackets in the next equations). The relation above comes from the definitions of G and of H:
The partial derivatives of these functions are
We have seen that the internal energy ∂U=∂q+∂W-(∑iNi∂μi) and that ∂S=∂q/T and that ∂W=-p∂V. As a result
The chemical potential is thus the variation of free energy of Gibbs with regard to the variation of concentration of one specific species, keeping the other parameters (p, T and the concentrations of the other species) constant.
We also define the partial molar volume
that is useful for gases. From the law of perfect gases,
That leads us to the expression of the chemical potential for a gas
In solution, the formula is very similar
We know that
So we can find the expression of ΔG as a function of the pressures or of the concentrations of the species:
Yet, this is only true for ideal solutions and gases. Normally, we have to consider the activity a of each molecule
γ is equal to 1 for ideal solutions but may vary if the concentrations increases, representing the increase of interactions between species. However, the activity a of solids is equal to 1.
Third principle
The entropy of a perfect crystal at absolute zero is exactly equal to zero.
The third law provides a reference for the entropy of any pure crystal (and therefore to anything) at any temperature. A perfect and pure crystal is a crystal the arrangement of which is perfectly organised: there is no flaw, the matrix is regular and it is composed by one species. At the absolute zero, i.e. zero Kelvin, the state of the system is at the minimum possible energy. This state is unique as a result of quantum mechanics and it is indeed the case: there is only one way to place the atoms in a perfect crystal. The amount of possible configurations is thus 1, leading to an entropy equal to
If a base state is degenerated, i.e. there are several states of same energy, there are several different possible configurations Ω and the entropy is not equal to 0 but is very close to it. As soon as there is a flaw in the crystal, the amount of possible configurations increases. As a result, the entropy increases. This variation of entropy is synonymous to an variation of energy as told by the second law
The temperature rise dT due to the heat ∂Q is determined by the heat capacity (cp ≈ cV) of the crystal
Consequences of the third law
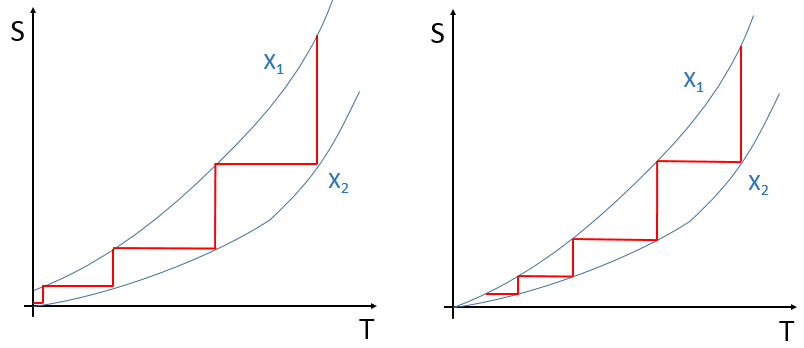
A first consequence of the third law is that T=0K cannot be reached by any number of finite processes. It is due to the fact that there is only one state of minimum energy. If we tried to change a given parameter in a controlled way so that the energy decreases each time, it would take an infinite number of repetition to reach the absolute zero. It is a bit similar to the problem of the bullet: If at each period of time a bullet moves from half of the necessary distance to reach its target, the target will be approached but never reached. If several states were allowed at T=0K, it would be possible to switch the parameter to obtain one or the other state: one value of the parameter approaches the system from one state and the other parameter approaches the system from the other state. With only one target, we cannot switch from one value of the parameter to the other and reach the final state. We can visualise this like this:
A second consequence is that the heat capacities cp and cV tend to zero when T tends to zero.